Some architects herald the adoption of freeform curvilinear design and the utilization of computer-aided manufacturing as cutting edge, or even avant-garde. While it may be so within the confines of architectural design and discourse, there is, in fact, nothing essentially new about these technologies. In comparison to our counterparts in other fields, architects are, in fact, behind the curve. This article seeks to addresses this deficit of knowledge by summarizing the ingenious development of the computational curve, which occurred in other industries. It’s a fascinating story. Any designer who draws curves with only a few clicks of the mouse, may benefit from knowing how this technology – which we now take for granted – was developed.

The need to compute curved 3D surfaces with the assistance of computer numerically controlled (CNC) manufacturing was the very impetus for the first computer aided design (CAD) software over half a century ago, principally developed in the automotive and aerospace industries. Digital animators in the entertainment industry developed methods of intuitively smoothing topologically ambiguous forms. These solutions have migrated into the software tools architects use today. However, the history of curvature obviously long predates the computational era. It extends back to our early ancestors’ most primitive endeavors to bend natural materials to human will.

Curvature has always played an important structural role in buildings, evident in the Roman arches and domes that remain standing to this day. Curved form has run throughout architectural history, extending the our modern era – of which the catenary vaults of Antonin Gaudí, Félix Candela’s thin shell roofs, and Frei Otto’s tensile hyperbolic paraboloids are notable examples. Curvature has also inflected architecture with aesthetic refinement. For example, the use of entasis in Greek antiquity made columns appear to bulge under the strain of their load. After enjoying a relatively brief heyday in the Modernist 1950’s and 60’s, curvilinear architecture had ebbed to an undercurrent by the late 20th century, navigated by only a few eccentric visionaries. Frank Gehry’s iconic Bilbao Guggenheim museum (1997) changed all this. It heralded in a renaissance of curvaceous formalism and broadcasted the arrival of the contemporary digital architectural era to mainstream culture. It is telling that the NURBS (Non-Uniform Rational B-Splines) software – specifically, Dessault’s Catia® – used to conceive and coordinate construction of the museum’s undulating titanium skin was technology originally developed for designing fighter jets. Both Catia’s and NURBS’ roots can be traced back to Paris in the 1960s, where the computational curve was invented nearly simultaneously in two rival car factories. The term ‘spline’ – synonymous today with the computational curve – can be traced back much further into the early history of naval architecture, where it originally referred to a flexible strip of wood used to scribe the smooth cross sections of boat hulls – a technique called lofting, practiced since antiquity. It’s no coincidence that these terms remain in the computer modeling lexicon we use today.

Given the current vogue for freeform surfaces in architecture, as well as the wider prevalence of ergonomic forms in our daily lives – from toothbrushes to bike helmets – it seems surprising so little historical research (architectural or otherwise) has been collected regarding the geometrical underpinnings of 21st century computer-aided design. From the commonplace to the sublime, the spline delineates contemporary aesthetics (curved and straight) because it is the lingua franca of the design, engineering, and manufacturing industries. Yet its history remains under-appreciated and its technical underpinnings are generally not comprehended, even by eager adopters, who tend to take modern 3D software’s point-and-click facileness for granted.

Most accounts of early computer aided geometric design (CAGD) come from (mostly academic) books and papers, written for a highly technical audience within that field. Apart for one or two notable exceptions,ⁱ historical developments are presented as an evolution of mathematical equations, without much commentary as to their wider importance for humankind. These texts assume a sophisticated knowledge of advanced mathematics, which, sadly, this author does not possess. Thus, this effort to unravel the story of the spline may be the first nontechnical account written for the intuitive understanding and benefit of the user base – ie. designers. For architects, specifically, it’s worth familiarizing ourselves with the spline’s influence over the wider field of design so that contemporary architectural developments can be placed within a more historically and technically grounded context. Demystifying the curve, I hope, will help to develop a better appreciation – perhaps even a connoisseurship – of what has now become a de facto architectural tool: the spline.

From Bows to Boats – the Mechanical Spline

By the Mesolithic era, humans had learnt to fashion bows in order to hunt game. As a hunter draws the bow, its two tips are pulled closer, producing greater curvature. As the timber is deformed, it stores elastic potential energy as the wood tries to regain its original straightness. Wood’s natural elasticity allows trees to grow tall, yet bend without snapping in the wind. When a length of wood is flexed, the material on the concave side goes into compression while the convex side is under tension. In bending, these forces distribute themselves as evenly as possible throughout the length of wood, producing a optimally smooth curve.

Evidence of Mesolithic settlements, as well as numerous indigenous cultures, show primitive shelters were constructed in a similar fashion.ⁱⁱ Excavations reveal where saplings were driven into the ground, forming the base perimeter of dwellings. The tops of opposite poles were lashed together to form an overall dome-like latticework and covered by animal hides, bark or grass. (The native american wigwam is a more recent example.) The elasticity of each timber pole produces an arch – an optimal structural shape in compression, imparting strength and rigidity to the crude dome (see Figure 1).

Since ancient history, humankind has harnessed the inherent utility of curved objects, not only to satisfy his basic needs for food and shelter, but also for transportation. In a crude sense, a boat is the simple inversion of the aforementioned primitive hut. When the skin or other outer covering is made watertight with tar, one has a basic (albeit unstable) dingy. The design and construction of boats has been subsequently refined over millennia, streamlined by the dynamics of water, wind, and speed.

The Romans used full-scale wooden templates, or moulds, to generate the variable wooden ribs that reinforced their ships’ hulls.ⁱⁱⁱ The curvature of each successive rib morphs along the length of the keel (see Figure 2). When covered with planking, these skeletal ribs underpin the smooth contours of the hull. The curved profile of each rib was traced from a reconfigurable master template, consisting of piecewise arced and straight wooden pieces. Graduated marks on the template indicated where the pieces were to be repositioned for each successive rib. Using this early parametric method, a fleet of identical ships could be manufactured from a single template.

The practice was refined throughout the northern Mediterranean well into the 1700s.^iv As shipbuilding evolved from a craft to a science, drawings replaced full-scale wooden templates. The practice of plotting the patterns for ribs and keels became known as lofting since the attic above the workshop was the only dry unobstructed floorspace large enough to accommodate the 1:1 setting out process. The long curves were scribed onto the timber from a thin flexible strip of timber or steel, called a spline (see Figure 3). The spline is bent and held in place on a flat surface by a series of three or more hooked metal weights, called ducks. An optimally smooth, attractive, and mechanically sound curvature is guaranteed by the uniform distribution of stress throughout the long elastic spline as it tries to regain its original straightness. This quality of even smoothness is known to shipbuilders as “fairness” and is championed because it minimizes the vessel’s drag in the water. By the 1600s, European shipbuilders had begun to rely on smaller scaled drawings for design and contractual documentation. Hand-held mechanical drafting splines were invented to trace the overlaid orthogonal projections and cross sections which set out the ship’s critical underlying geometry. However, when translating drawings into full-scale parts, boatbuilders still employ the traditional lofting technique using a spline to this day.

Shipbuilders were not the only designers using splines around this time. The great Italian Renaissance architect Andrea Palladio describes in his famous treatise, The Four Books of Architecture, how he used a flexible ruler to trace the graceful swelling profile of classical columns.^v More conventionally, designers came to rely on wooden or steel templates to trace smooth curves. The German kinematician and geometer Ludwig Burmester published a standardized set of curve templates (see Figure 4) around 1900 (Lueger, 1904) .^vi These became widely known as French Curves. Each scroll-like template is an amalgamation of assorted mathematical curves. To use it, a draftsperson finds a close fit along the edge of the tool and repositions it to continue drawing tangential curves. Until the advent of modern computing, French Curves were the tool of choice for architects when drawing any irregular smooth object.

L’Automobile – Inventing the Computational Spline

The birth of modern computer aided design (CAD) can be traced back to French automaker Citroën.^vii By the mid-1950’s the brand had cemented it’s name as a leading global innovator. The DS was Citroën’s flagship model, packed with avant-garde technology, such as a self-leveling hydropneumatic suspension system to lift the “Goddess”— DS is pronounced the same as the French word for Goddess: déesse — allowing her to float effortlessly above France’s bumpy post-war roadways.

When it was revealed at the 1955 Paris Motor Show, the radically curvaceous DS appeared poised to drive France into the Space Age. The philosopher Roland Barthes (1957) described it as a superlative object as if “fallen from the sky”.^viii The body was, in fact, styled by an aeronautical engineer, André Lefèbvre, around the time Citroën began using wind tunnels in its design. The DS also had artistic leanings thanks to the hands of Italian sculptor Flaminio Bertoni, who collaborated in shaping its exotic form.

The DS was not merely a product of streamlining. It was also the first mass-produced unibody automobile, meaning that the rounded metal skin supported structural loads rather than relying on any internal steel frame. More than fifty years after its launch, the DS is still idolized (particularly by designers) for its idiosyncratic functionality resolved within an elegant form. Barthes summarized this poetic integration quite succinctly:

“(the DS) excites interest less by its substance than by the junction of its components. It is well known that smoothness is always an attribute of perfection because its opposite reveals a technical and typically human operation of assembling.”

Yet the seamless assembly of groundbreaking automobiles, like the DS, presented Citroën with a critical challenge. Its solution would radically re-shape design and humans’ command of geometry and form.

In addition to full-scale blueprints, Citroën’s and other carmakers’ design process relied largely on physical models for conceptual development, as well as preserving, translating, and sharing the automobile’s geometry amongst various teams. The styling department began by crafting small-scale conceptual models of the new prototype. Curved surfaces had to be manually enlarged to full-scale by measuring points offset from these smaller clay mock-ups, then re-plotted onto a life-sized drawing board. The plotted points were interpolated using French Curves or flexible spline rulers to arrive at a best-fit approximation. Plywood cross sections would be cutout and assembled crosswise, creating a intersecting matrix formwork. The skeleton was fleshed out with clay and further sculpted to create a detailed master model of the car. In its final form, the master model would continue to undergo subtle shaping and refinement at the hands of skilled plasters before being frozen in its final shape, ready for production.

Designers, engineers, and machinists could refer to the master model’s geometry (or one of several copies of it) when designing parts and the tooling required to manufacture them. Extracting the relevant geometry and ensuring it fitted seamlessly within the overall assembly would entail similarly onerous techniques of translation. Several design iterations were required to finalize each part relying greatly on the workers’ skill, ingenuity, and subjective judgement. However, the often qualatative interpretation of the geometry introduced significant room for inconsistency, discrepancy, and human error. The company needed a common geometrical language to store each part in terms of numbers, rather than relying on time consuming and error prone process of manual replication.

By the late 1950’s, Citroën and its competitors had begun adopting – thanks to government support – early analog computers connected to machinery for milling the stamps and dies that repetitively press parts of the bodywork from sheet metal. These early CNC machines required coordinates be input in the form of holes punch along a paper tape to guide the tool’s path and direct the depth of travel as it excavated material.^ix Lines, circles, parabolas, and other regular geometrical functions could be accurately inputted from the designer’s blueprints into the machinery, but a reliable method for freeform curves – much less surfaces – did not exist.

In 1959 Citroën hired Paul de Faget de Casteljau, to find this missing link. Fresh from his PhD studies, the young mathematician was tasked with devising a system of equations, so that shapes could be reliably stored and communicated amongst the designers and engineers, as well as input into the primitive computers that drove the new milling machines. De Casteljau struggled with the problem at first. His epiphany occurred when he realized that, rather than defining a curve by points along its length, it could be accurately plotted and controlled by manipulating a few points around its vicinity.^x When these points of influence were moved, the curve would follow, similar to the intuitive response of moving the weights that anchored the boatbuilder’s spline.

De Casteljau’s algorithm was a method of recursively evaluating an already existing mathematical expression, the Bernstein polynomial. The formulas describing this differential geometry may be mathematically advanced, but they can be intuitively understood (see Figure 6). The points of influence are connected to form control lines, or what de Castelaju termed a courbes à pôles. Points are created at proportional distances (ie. 2/3rd of the way) along each of these poles. New lines connect these points and, once again, a new set of points is created at the same proportional distance along them. New poles and points are generated until a final point remains. Mathematically, the process can be repeated at all intervals (ad infinitum, thanks to computation), plotting a smooth course of curvature.

Upon making his discovery in 1963, de Casteljau immediately rushed to the workshops to set about recreating the the hood of a 2CV from mathematical data alone, only to find that the modelers were unwilling to cooperate. De Casteljaus’ theory was also met with incredulity elsewhere within Citroën. How could a mere ten lines achieve what others before him had unsuccessfully attempted in sixty pages of equations? The modelers scoffed at de Casteljau’s poles, which seemed overly intuitive, claiming that anyone could have invented them. How could this be a worthy method? If valid, it threatened to replace the black arts of auto production with cold hard computation and, in doing so, make the influential role of master modelers obsolete. The young mathematician did eventually convince the company that his elegant algorithm was the ultimate solution they had sought by replicating ever more complex auto parts from numerical data, culminating in a full body model of the GS. Welcome or not, the computational curve had arrived.

Citroën enforced strict secrecy surrounding de Casteljau’s breakthrough and his findings went unpublished for eight years. It was roughly fifteen years before he was personally credited with his discovery. However, confidentiality did not prevent his counterpart at Citroën’s direct competitor, Renault, from learning of his achievement, albeit not the secret algorithm itself. That man would go on to independently recreate de Casteljau’s achievement, and in doing so, make his own name synonymous with the curve in its computational form. Renault’s Head of Design at the time was an engineer named Pierre Bézier.

In the 1960s, Renault faced identical challenges to Citroën.^xi Chiefly, how to transform curves from the drawing board into a reliably accurate mathematical language compatible with early digital computers? Renault also found the process of enshrining the geometry of each car in a master model and transferring it to the various parts fraught with internal disagreements, retouching, delays, and expense.^xii Bézier knew what Citroën had discovered, but he did not how it was done. His method of tackling the problem was quite different from Casteljau’s, but the end result was analogous. Early on, Renault’s man relied on empirical observation and his imagination. In addition to curved drawing tools, his department had begun to use simple mathematical constructs, equivalent to cartesian grids onto which regular mathematical curves – arcs, ellipses, and parabolas – were plotted. Once formulaically enmeshed within the two-dimensional grid, these curves could be morphed by mathematically deforming the grid around them. But when dealing with more complex real-world curves in 3D – the edge of a door panel, for example – it was impossible to apply this method. The fundamental problem remained: how to define freeform curves that bend and twist within three-dimensional space?

Bézier envisioned a room in which a flexible metal extrusion is suspended between the floor, walls, and ceiling by a rig of springs, weights, and pulleys. Although this imaginary contraption was impractical, it proved conceptually useful. A 3D parametric curve can be expressed as the intersection of two cylinders (see Figure 7). The center and radii points of these cylinders correspond to vertices of a cube, encompassing the curve within. Bézier found that by mathematically transforming the outer cube, he could control the distortion of the curve within. As he developed the idea, he found only three connected edges of the cube were needed to construct his irregular curves, and that he could formulate them as polynomials. Making more complicated curves, simply required more interconnected lines.

By 1966, Bézier had achieved the same result as as de Casteljau’s courbes à pôles, but unlike his competitor, Renault allowed him to publish his findings. Bézier was credited with inventing the computational curve that bears his name. Later, the two mens’ methods would be mathematically reconciled and both are widely credited with the discovery. The subsequent integration of their geometry into mainstream computing has directly benefitted every design field. For instance, graphic designers will recognize Bézier curves as the tool that enables them to draw smoothly by intuitively clicking and dragging vertices in software applications like Adobe Illustrator. In fact, Adobe’s decision to base their Postscript language (as well as Apple’s True Type) on cubic Bézier curves lead to the desktop printing revolution of the 1980s. It revolutionized typography. The average computer user is probably unaware that Bézier curves so elegantly describe the curved outlines of fonts, allowing text to be printed smoothly at any scale whilst consuming negligible storage space (see Figure 8).

Of course a car, being a three dimensional form, is not merely defined by a series of composite curves. Sheet metal body panels, for example, vary in their curvature at almost every point. Defining only the perimeter curves of each part does not describe its interior contours. To construct freeform surfaces, Bézier found it useful to refer to an old foundry technique, used to produce a variable surface on a casting. Sand is packed into an open-topped wooden box. Two of the opposing top edges of the box are curved so that when a wooden plank or template is dragged across them, it scrapes off the remaining sand to leave a smoothly curved surface.

Bézier surmised that if only one could modulate the shape of the template as it was being dragged across the guides, any curved surface could be formed in one steady sweep. Again, he formulated a mathematical construction of his hypothetical tooling (see Figure 9). Bézier employed his newly-discovered curves to delineate the two curved outer edges of the box. Between these, another pair of curves is inserted to yield four unique curves in one direction. Points at equal proportion along each of the four curves can be connected across to create another curve running in the transverse direction (analogous to the template being dragged between the outer rails). As the transverse curve travels across the four guide curves, the shape morphs along them and defines the contours of a smooth freeform surface. The matrix of control points forms an underlying control net with vertices that can be easily moved to shape and sculpt the resultant surface. Like de Casteljau, Bézier also experienced initial resistance to his ideas from top management, who told him, “If your system were that good, the Americans would have invented it first!”^xiii In fact, the Americans had not yet done so, but General Motors would subsequently play an important role in the development of a computational spline.

GM’s predicament at the time was similar to that of the French automakers. By 1959 the company had acquired numerically controlled milling machines, but lacked a mathematical language to input the complex shapes of its streamlined cars, with all their space age tail fins and voluptuous styling.^xiv Carl de Boor, a researcher at GM, devised a recursive method for evaluating B-splines, a fairly obscure mathematical function, first conceived in the 1940s (although the mathematical development can be traced back to the nineteenth century), by I. Schoenberg for smoothly – albeit laboriously – plotting graphs of actuarial data.^xv At GM, B-Splines’ powerful flexibility and superior control was truly unleashed through the recursive speed of computation.

Like a Bezier curve, the B-spline is controlled by a group of linked control points. The B in B-spline stands for its multiple basis functions. Each defines an overlapping portion along the spline. The overlapping sub-curves are trimmed and tied together at uniform intervals, fittingly called “knots”. Each basis’ segment shares all but one of its control points with those adjacent (see Figure 10). This property blends them smoothly together into a spline. Moving one of the polygon’s control points affects only the segments closest to it, because its influence falls off to zero according to the base functions’ bell curve formula. This imparts local control over a region when control points are shifted, but without altering other parts of the spline. The B-Spline, therefore, is more accurate and controllable, just like its physical namesake. It is also possible to close the B-spline, creating a tangential loop. Mathematically, the B-spline was later discovered to be a generalization of Casteljau’s algorithm, including it as well as a greater range of geometries. It also implicitly includes the Bezier Curve as a special case.^xvi

Aerospace – Finding a Universal Language

Curvature is nowhere more critical than in the aerospace industry, where the laws of aerodynamics require designers to draw smooth curves, manufactured to tight tolerances. Naturally, early aeronautical engineers adapted long-established naval techniques to loft airplane wings, and fuselages. Before the widespread advent of CAD, draftsmen used flexible drafting splines and French Curves to draw curved geometry. But aerospace designers also resorted to other classical geometrical constructions that dated back to the the 1600’s. One was the conic. By rotating a plane, its line of intersection with a cone produces a range of circles, ellipses, parabolas, and hyperbolas (see Figure 11). Designers could draw the cross sections of a fuselage by chaining together tangential conic segments. From four points a practiced draftsmen could, using only a ruler, construct such a conic curve through a simple, yet laborious, process of repetitively intersecting and subdividing straight lines – a process not dissimilar from string art.^xvii However, smoothly connecting their plotted points still required the help of French Curves to fit them as closely as possible into one smooth curve.

During World War II, lofting airplanes (a good deal of terminology and techniques were inherited from shipbuilding) in hangars became a strategic liability. A single bomb could obliterate the master templates of a tactically vital airplane.^xviii Furthermore, drawings could be stolen or photographed and were impossible to encrypt. Roy Liming was an aerospace engineer during WWII, working for North American Aviation (after the war it became a part of Boeing) which manufactured fighter planes, like the Mustang. By converting parametric conics into algorithms, each plane’s critical geometry could be stored numerically in tables, rather than vulnerable physical blueprints. This became advantageous since numbers could be stored safely and were easily transmitted. Furthermore, they offered, efficiency, accuracy, reproducibility, and security.

After the war, private aerospace contractors, like Boeing, continued using Liming’s formulas. By the mid-1950’s, the company had developed its own design software for drawing fuselages based on Liming’s conics. However, it was still not uncommon to see draftsmen using wooden splines at their desks a decade later.^xix As CAD became more widespread, departments bought or developed their own specialized software to handle the different component geometries of the aircraft;^xx for example, wing design required continuous splines for aerodynamic analysis. Throughout the 1960-70s, the limitations of such methods had become more acute when designing, manufacturing and assembling various parts of the aircraft.

Boeing was a microcosm of the fragmentary innovations in early computer-aided design, which tended to vary according to the specialized needs of each technical industry or department. Faced with systemic incompatibility problems, the company needed to standardize its geometry in order for teams to collaborate efficiently by sharing and coordinating design geometry and software. In 1979 the company appointed a group of mathematicians to select standard representations for eleven curve forms – everything from lines and circles to Bézier curves and B-splines.

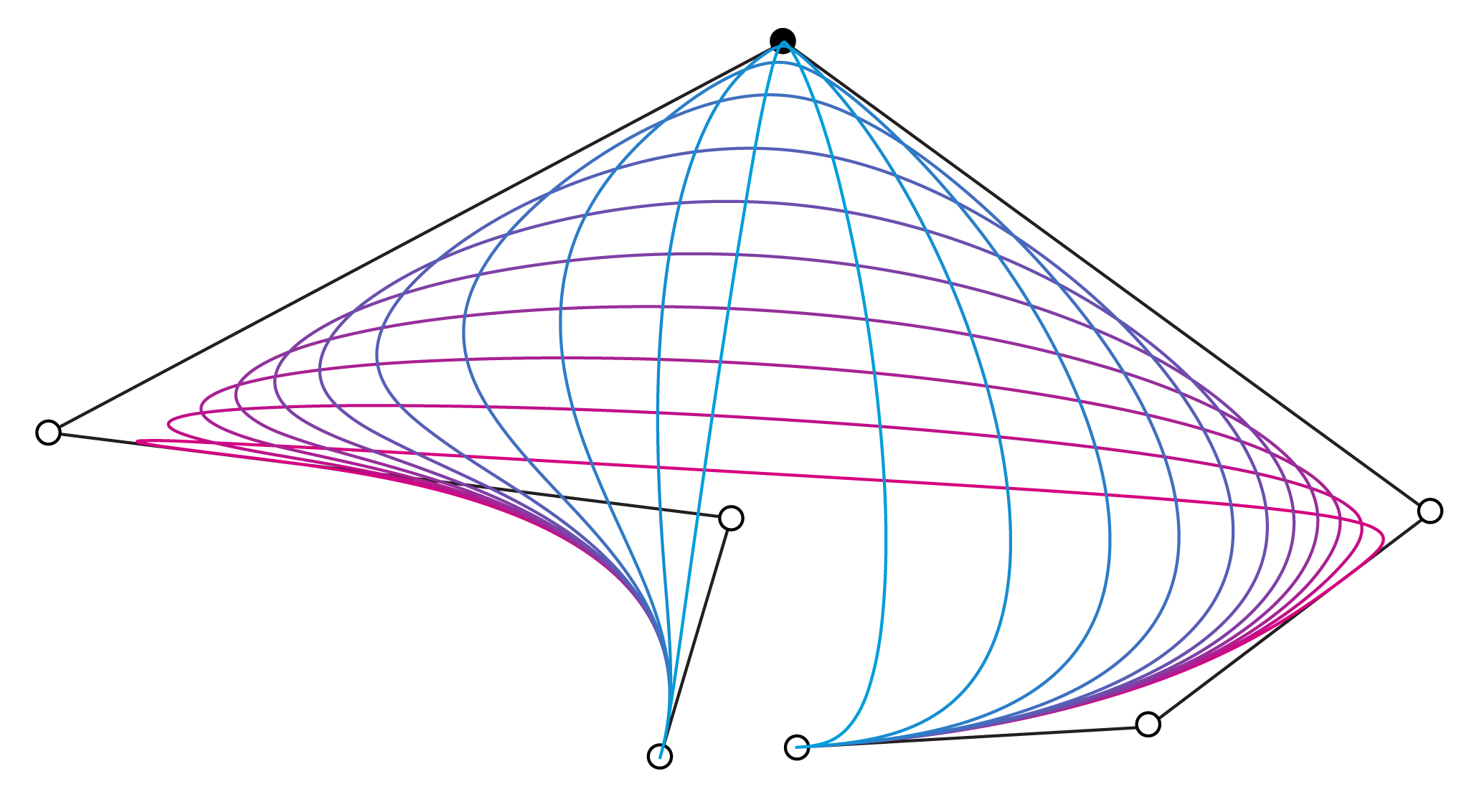
The team soon recognized their goal should be a single all-encompassing curve representation, rather than eleven separate ones. They devised rational Bézier curves – rational meaning that the weight ratio of each control point could vary (see Figure 12). Variable weighting made it possible to draw conics precisely, as well as cylinders and planar parts. A few years earlier, PhD students of S. Coons at the University of Syracuse had expanded the B-Spline model to be both rational and non-uniform. Boeing incorporated the non-uniform B-Spline – whose knots are non-uniformly spaced along the curve – into their new representation. This property could introduce sharp corners within the spline by placing multiple knots in one location, effectively pinning the curve into a corner at that point. The team at Boeing found a way to combine rational Bézier curves and non-uniform B-Splines. By 1981, they had devised a new geometrical model that could encompass all of Boeing’s needs, from simple lines and circles, to the most complex free-form curves and surfaces. This new mathematical representation was called Non-Uniform Rational Basis Splines, or NURBS for short.

NURBS were quickly adopted by engineers and designers. In the same year, the Boeing researchers convinced the Initial Graphics Exchange Standard (IGES), a consortium of industry and government bodies, to adopt NURBS. IGES became the American Standards Institute (ANSI) CAD standard, making it the lingua franca for digital exchange between CAD software. Today, organizations can use NURBS to draw fuselages, wings, and almost any design component within the same software, greatly advancing integration and collaboration between design teams, software platforms, or companies. Without NURBS as a shared geometrical language, it would be impossible to imagine modern manufacturing – be it auto, aerospace, or just about any other product – with teams of specialist subcontractors and suppliers all working cooperatively on shared 3D software models with great precision.

Animation – Freedom From Topology

As computer aided design matured, the field of computer animation was still in its infancy. One upstart studio in particular, Pixar, pioneered CGI (computer graphics imaging), releasing its first feature-length film, Toy Story, in 1995. Pixar’s animators painstakingly modeled the film’s characters and props using NURBS geometry. However, modeling and animating characters smoothly with NURBS surfaces proved extremely difficult. NURBS is limited to surfaces that are topologically rectangular, such as distorted planes, cylinders, spheres, and toruses (the volumes are actually produced by trimmed and curved rectangular surfaces, in which one or more edges are joined to form an enclosed shape). But organic forms are rarely so simple. Their topology is arbitrary, with numerous holes, creases, and protrusions. To recreate these types of objects with NURBS demands a piecemeal approach.

Each of Toy Story’s characters – like Woody, the cowboy doll – was created from a patchwork of individual NURBS surfaces. Each had to be skillfully trimmed and shaped to create the illusion of blending seamlessly into the next. The process was expensive, time-consuming and error-prone. Making matters worse, when animated, the characters’ seams had an annoying tendency of bulging awkwardly or splitting open, demanding hours of costly manual frame-by-frame correction. In their next animated short, Geri’s Game (1997), Pixar tried it a different way, using subdivision surfaces for modeling the characters.^xxi

Subdivision surfaces built upon research, completed 16 years earlier, by Edwin Catmull (current president of Disney and Pixar Animation Studios) and Jim Clark (founder of Silicon Graphics), examining the possibilities of recursive uniform B-Spline surfaces.^xxii Subdivision surfaces enabled modelers to sculpt their characters from a single 3D form, much like a sculptor begins with a lump of clay. Underlying the subdivision surface is a control polygon, resembling a coarsely facetted version of the smooth object. The computer repetitively subdivides the vertices of the polygon by approximating new vertices between them. The polygon is repeatedly subdivided until the facets are so fine they disappear into a smooth surface (see Figure 13). With the increased processing power of modern computers, recursive subdivision appears instantaneous. The user can simply move the vertices of the underlying control mesh while simultaneously viewing the resultant changes in the smoothed form.

By using subdivision surfaces, Pixar’s animators could more easily mold characters whose skin and clothing did not conform to a simple square topology. Characters could be reliably animated to run, jump, and crouch without literally coming apart at the seams. Additional algorithms allowed the surface to be creased by marking certain edges of the control polygon as ‘hard’. The edges of fingernails or eyelids could be articulated and detailed without breaking continuity with the surrounding skin. Pixar now relies on subdivision surfaces in all of its animated films and the technology has become a standard feature within modeling and animation software.

Animators’ migration to subdivision modeling was their industry’s point of technological divergence from design. Designers and engineers continue to favor NURBS because it offers the precise control over curvature they need for accurately manufacturing real-life products. Animators – who need only be concerned with how a moving shape appears on screen – have gladly relinquished this control in favor of the artistic flexibility and speed offered by subdivision surfaces. Software targeted at aerospace, automotive, and product design markets offer a set of sophisticated NURBS-based parametric modeling tools that can look and behave somewhat like subdivision surfaces, but are still fundamentally limited by their underlying NURBS topology.

The history of the computational spline is – in a strictly mathematical sense – a record of successive mathematical generalizations (see Figure 14). In other words, each succeeding geometrical representation has included and expanded on one or more preceding ones, with the goal of greater inclusivity. For example, NURBS can represent earlier conics, Bezier Curves, and B-splines, as well as simple circles, arcs, and lines. The next logical step is a bridge between NURBS and subdivision surfaces, as they share common a common ancestor in B-Splines. This important reconciliation appears to have recently occurred in the form of T-splines.^xxiii Although still not a perfect generalization of NURBS in purely mathematical terms,^xxiv T-splines seems to be on the cusp of widespread commercial implementation throughout a range of industries. They are forward and backward-compatible with NURBS, meaning T-Splines can integrate within existing CAD/CAM workflows. Beginning life as a proprietary plug-in to McNeel’s popular NURBS modeling software Rhino, the developer, T-Splines Inc., was bought in 2011 by Autodesk Inc., which now dominates the CAD and CGI software fields across numerous market sectors.^xxv T-Splines promises designers a superior 3D toolset by merging the accuracy and control of NURBS with the intuitive sculptural interface and topological freedom of subdivision surface modeling.

Subsequent developments will ultimately enable the designer – or perhaps anyone for that matter – to intuitively mold objects without being constrained to the technically tedious and inflexible process of blending disparate surfaces, constructed from fixed construction geometry. Once this occurs, expect to see a greater level of integration, articulation, and refinement of freeform designs. 2013 saw 3D printing go mainstream. This trend, along with the ever-increasing affordability of computation and a proliferation of software choices – particularly free and open-source alternatives – will democratize complex geometry, expanding 3D software’s user base beyond specialized industries and their trained software operators. Will this trend lead to haptic computer modeling interfaces, as tactile and accessible as folding cardboard, molding clay, or bending a strip of wood? The latest generalization of the spline brings mankind another step closer to complete mastery of form.

About the Citroën DS, Roland Barthes spoke of “a new phenomenology of assembling, as if one progressed from a world where elements are welded to a world where they are juxtaposed and hold together by sole virtue of their wondrous shape, which of course is meant to prepare one for the idea of a more benign Nature.” The geometrical singularity Barthes envisioned is rapidly approaching.

Architecture – Descendant Form

The realistic lighting, materials, and rendering quality offered by animation software has proven superior to the visualization achievable using standard CAD packages. For this reason, architects have appropriated animation software as a design tool since the 1990’s. An early epicenter was (not coincidentally) Southern California, where a chief proponent of Hollywood special effects software, Greg Lynn, coined the term blob architecture.^xxvi (The movement later elicited a more pejorative portmanteau: blobitecture.)^xxvii In addition to photorealistic rendering capabilities, the animators’ toolset – specifically, subdivision surfaces – has offered adventurous designers the sculptural freedom to easily develop designs unconstrained by Euclidian topology. As a result, shiny amorphous forms have been pouring out of architecture schools ever since, accompanied by a weighty body of conceptual discourse to substantiate these new formal approaches.

Despite the increasing ease of producing exotic digital forms in the computer, a challenge persists for practicing architects and engineers: chiefly, how to feasibly construct such complex geometry? In this regard, architecture, engineering, and construction (AEC) have made tremendous advances, yet the state of the art lags behind manufacturing, where curvature is de rigueur. But that’s understandable. Buildings are typically designed as one-offs, not robotically mass-produced offshore by their thousands in hermetically controlled factories. The AEC industry is not as consolidated as automotive manufacturing, where only a few companies control a vast market, spending R&D budgets in the billions of dollars to develop singular products. The challenge that motivated early pioneers like Casteljau and Bézier – using computation to rationalize irregular forms into discrete components for fabrication by early computerized machine tools – is still currently unfolding in architecture. For architects who design fluidly with subdivision surfaces, a critical challenge lies in rationalizing the boundless topology of conceptual forms into discrete components that can be fabricated and assembled. BIM (Building Information Management) and other parametric NURBS-based modeling software organize 3D building geometry through logical relationships, promising to make 2D documentation obsolete by integrating fabrication and design within a common 3D platform. BIM tools have so far lacked subdivision surface modeling because of its incompatibility with NURBS, but perhaps not for much longer.

Curviform architecture (à la Hadid, Gehry, etc) is still far from mainstream. It requires specialized software knowledge to design (let alone rationalize and build) such expressionistic works. Despite being designed with computers, buildings styled by curve-loving celebrity architects require additional design labor and 3D CAD expertise, especially when compared with more conventional flat and orthogonal alternatives. Since they are difficult and, typically, more costly to build (and therefore, reproduce and immitate) they are more unique and (arguably) more valuable. However, the barriers to entry into this elite field – a technical knowledge of how to generate and manipulate complex geometry – are constantly lowering, with evermore powerful and user-friendly 3D modeling interfaces. These abstract complex mathematical spline operations by recoding them into a set of tools that mimic our real-world experiences of making: lofting, trimming, extruding, lathing, sweeping, welding, sculpting, etc. T-splines and other potential generalizations of subdivisional surfaces and NURBS will remove a significant barrier, perhaps eventually trivializing the design and construction of freeform buildings.

Rational proportion and regularity lay at the foundation of classical aesthetics, predicated on empirical definitions of beauty and natural order. These were codified, repeated, and reinterpreted throughout various neoclassical revivals. Splines also possess quantifiable parameters that can validate their aesthetic pleasingness. Order, tangency and fairness are technical terms, quantitatively describing the mathematical continuity of the spline. These variables control distortions in the rate of change in curvature, recognizable to the human eye as bulges, dents, or creases. Instinctively, we are highly attuned to perceive these qualities – say, when admiring the twisting torso of a Greek statue. Automakers still rely on the human eye to scrutinize bodywork, by passing the car through a tunnel of strip lights and verifying the reflections are smooth before it rolls off the assembly line.

Splines and flowing surfaces don’t easily lend themselves to discrete rules and pattern books, because they seem to lack finite dimensions – edges, corners, sides, roof, walls, etc. We also find it hard to fit words to curvature when discussing architectural design. Performance-driven industries like naval, auto, and aeronautical design, better understand curvature for its quantifiable (not to mention highly marketable) benefits. Studying the path of the spline’s history provides a fuller perspective on contemporary developments in architectural form, as part of a continuum of humanity’s geometrical advancement. Each new iteration of the spline has reconciled divergent models, stepping ever closer to a universal geometry. As computation continues to erode architecture’s formal constraints, curvature will soon become unremarkable, like so many of the molded plastic products we consume daily already are: disposable razors, computer mice, bottles of detergent, watering cans, plastic toys, etc, etc… Stripped of geometrical pretense, architects may champion such splinear forms simply for their subtle sophistication, structural elegance, and (dare I say it) shear beauty.